The Galileo Energetic Particles Detector
Galileo EPD Handbook
Chapter 1. Instrument Summary
Charged Particle Response of Magnetic Deflection System for Galileo Jupiter Orbiter (draft) (continued)
Magnetic Field Model
The magnetic field is due to a uniform magnet lying in the x-y plane with its center at the origin; its integral form [7] is given by

where σM is the surface density of the magnetic pole strength. M is the magnetization, which is constant, and its direction is the same as the normal of the magnet. The factor M/c is converted to the normalization constant B0 which was used to fit experimental values of the field.
We obtained this three-dimensional, analytic expression for B(x,y,z) by evaluating the integral [8]. Its components are given in equations (5), (6), and (7). From these expressions, we constructed a complete analytic expression for the field provided by both separate magnets. The experimental measurements of magnetic field strength are only available in the middle plane (z=0.0) where, because of the symmetry of the sensor, Bx=By=0 should vanish [9]. The two magnets are placed in a pattern: one at the left tilted counter-clockwise by an angle, the other tilted at the right clockwise by an angle of the same degree. For this arrangement, the expressions of both magnets require a coordinate rotation as well as a translation of the coordinate system. These transformations are given in equations (8), (9), and (10).

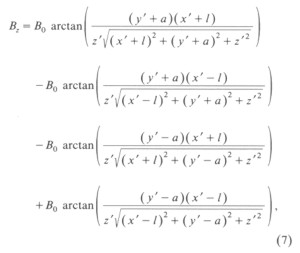
where a is the half-width of the magnet, l the half-length of the magnet, and B0 the normalization constant of the magnetization.
The rotation of the coordinates for the left magnet is given by
![]()
the rotation of the coordinates for the right magnet by
![]()
and the translation of the coordinates for both magnets by

where y'1 and z'1 are for the left magnet, y'2 and z'2 are for the right magnet, d is the separation between the left and right magnets, ψ is the angle by which the magnets are tilted, and x1, y1, and z1 are the distances shifted from the origin of the coordinates.
In addition, in order to simulate the actual measurements of the field, the two magnets are further divided into smaller pieces. For each of the smaller magnets, the rotation and translation were carried out. The magnetization values of all small magnets are obtained by normalizing the maximum field value from the experimental data. Figure 3 provides the distribution of the magnetizations of these magnets.
 |
Figure 3. Comparison between measurements and results of the model. Each curve corresponds to a different specific value of y (left) and x (right). |
The superposition of these small magnets (both left and right) results in the total field strength and gives a good agreement with the experimental data inside the sensor (see Figure 3). The magnetic field lines are computed and projected on both the y-z and the x-z plane as given in Figure 4. These field lines converge to the fringes of the magnets. The contour plots in Figure 5 demonstrate the expected variation of the field and show the divergence, and the curl of the magnetic field vanishes inside the sensor. The results show that the edges of the magnets are highly magnetized and the field is stronger where the two magnets are closer. The numerical results of the magnetic field of the magnetic field and the parameters used in the simulation are provided elsewhere [10].
Next: Methods of Calculation
Return to Galileo EPD Handbook Table of Contents Page.
Return to main
Galileo Table of Contents Page.
Return to Fundamental
Technologies Home Page.
Updated 8/23/19, Cameron Crane
QUICK FACTS
Mission Duration: Galileo was planned to have a mission duration of around 8 years, but was kept in operation for 13 years, 11 months, and 3 days, until it was destroyed in a controlled impact with Jupiter on September 21, 2003.
Destination: Galileo's destination was Jupiter and its moons, which it orbitted for 7 years, 9 months, and 13 days.







